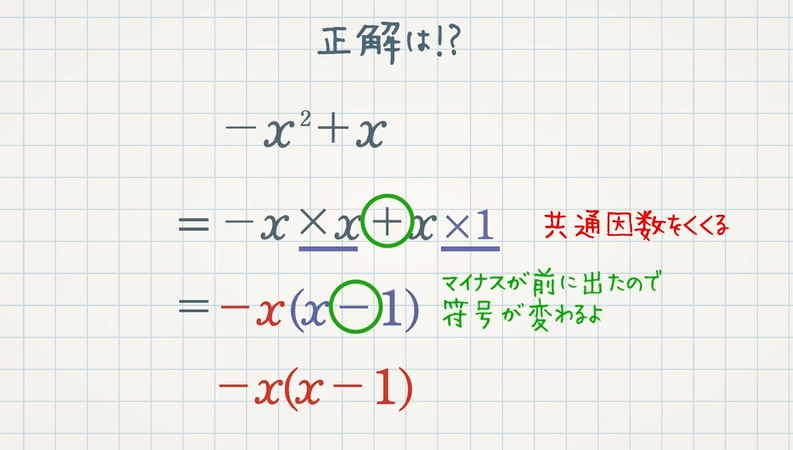
因数分解とは、多項式をいくつかの単項式や多項式の積の形で表すことでした。(展開を逆にみたものが因数分解になります)
各項に共通な因数をふくむ多項式は分配法則を使ってかっこの外にくくり出すことができます。
\begin{eqnarray} & &a\textcolor{blue}{{\rm M}}+b\textcolor{blue}{{\rm M}} ←\textcolor{blue}{{\rm M}が共通因数}\\ &=&\textcolor{blue}{{\rm M}}(a+b) \end{eqnarray}