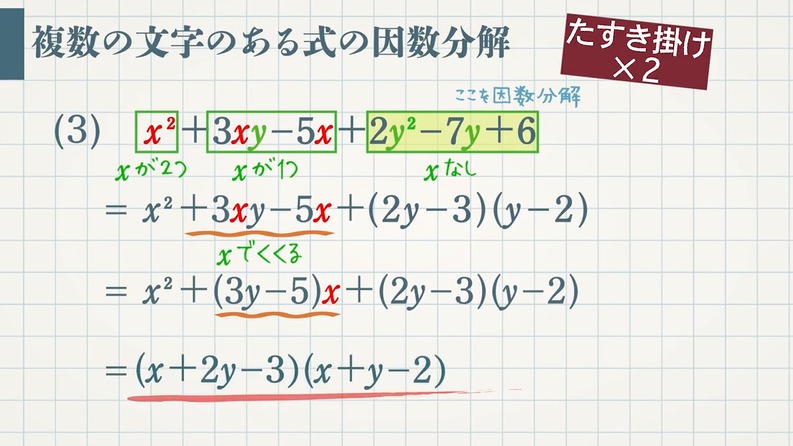
複数の文字のある式の因数分解のポイントは $2$ つです。

① 次数の最も低い文字に着目し、降べきの順に整理する。

② 次数でわけたグループごとに因数分解する

【例題】次の式を因数分解しなさい。

\begin{eqnarray} \textcolor{green}{(1)}&　&\textcolor{green}{a^2-3a+ab-b+2}　b について整理\\ &=&ab-b+a^2-3a+2　グループごとに因数分解\\\\ &=&b(\textcolor{blue}{a-1})+(a-2)(\textcolor{blue}{a-1})　a-1 を\rm Aに \\\\ &=&b\textcolor{blue}{\rm A}+(a-2)\textcolor{blue}{\rm A}　共通因数 \rm A でくくる\\\\ &=&\textcolor{blue}{\rm A}(b+a-2)　\rm Aを a-1 に\\\\ &=&\textcolor{red}{(a-1)(b+a-2)} \end{eqnarray}

\begin{eqnarray} \textcolor{green}{(2)}&　&\textcolor{green}{y^3-yz-x^2y+xz}　z について整理\\\\ &=&xz-yz-x^2y+y^3　グループごとに因数分解\\\\ &=&z(x-y)-y(\textcolor{blue}{x^2-y^2})　さらに因数分解\\\\ &=&z(\textcolor{blue}{x-y})-y(x+y)(\textcolor{blue}{x-y})　x-y を\rm  A に\\\\ &=&z\textcolor{blue}{\rm A}-y(x+y)\textcolor{blue}{\rm A}　共通因数 \rm A でくくる\\\\ &=&\textcolor{blue}{\rm A}(z-xy-y^2)　{\rm A} を x-y に\\\\ &=&\textcolor{red}{(x-y)(z-xy-y^2)} \end{eqnarray}

\begin{eqnarray} \textcolor{green}{(3)}&　&\textcolor{green}{x^2+3xy-5x+2y^2-7y+6}\\ &　&x,y どちらも同じ次数なので、並べかえなし\\\\ &=&x^2+3xy-5x+\textcolor{blue}{2y^2-7y+6}　たすき掛け\\ &=&\textcolor{blue}{x^2}+(3y-5)\textcolor{blue}{x}+\textcolor{blue}{(2y-3)(y-2)}　たすき掛け\end{eqnarray} 　　　

\begin{eqnarray} \\\\ 　&=&\{x+(2y-3)\}\{x+(y-2)\}\\&=&\textcolor{red}{(x+2y-3)(x+y-2)} \end{eqnarray}