文字式での表し方には決まった表し方があります。
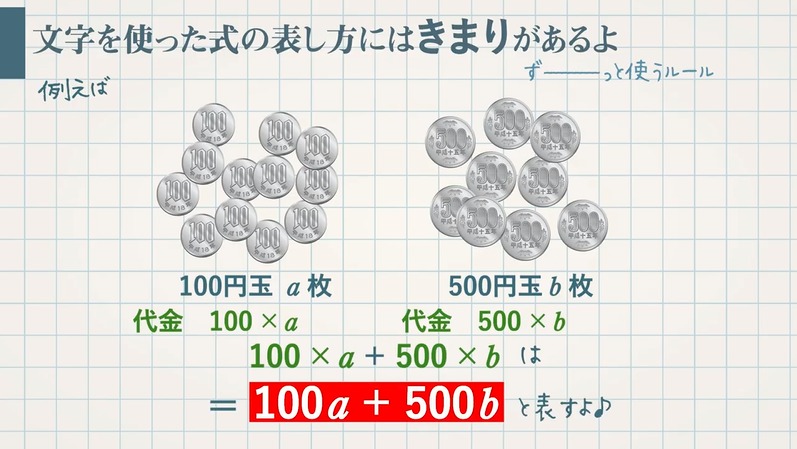
例えば、$100$ 円玉 $a$ 枚と $500$ 玉 $b$ 枚の金額の合計はというと、
$100$ 円玉が $a$ 枚なので、$100×a$ 円
$500$ 円玉が $b$ 枚なので、$500×b$ 円
よって、合計金額は $100×a+500×b=\textcolor{blue}{100a+500b}$ と表します。どういった決まりがあるのか、みていきましょう。
文字式での表し方には決まった表し方があります。
例えば、$100$ 円玉 $a$ 枚と $500$ 玉 $b$ 枚の金額の合計はというと、
$100$ 円玉が $a$ 枚なので、$100×a$ 円
$500$ 円玉が $b$ 枚なので、$500×b$ 円
よって、合計金額は $100×a+500×b=\textcolor{blue}{100a+500b}$ と表します。どういった決まりがあるのか、みていきましょう。

まずは、文字式の表し方の $4$ つの基本ルールを確認しましょう。
① 文字の混じったかけ算では、「$\textcolor{blue}{×}$」をはぶく
例 $a×b×c=\textcolor{blue}{abc}$
② 文字と数の積(かけ算)では、数を前にかく
例 $a×2=\textcolor{blue}{2a}$
③ 同じ文字の積は、累乗を使う
例 $x×x×x×y×y=\textcolor{blue}{x^3y^2}$
④ わり算では、「÷」を使わず、分数の形でかく
例 $a÷b=\textcolor{blue}{\frac{a}{b}}$
基本ルール以外の細かなルールを問題で確認していきましょう。
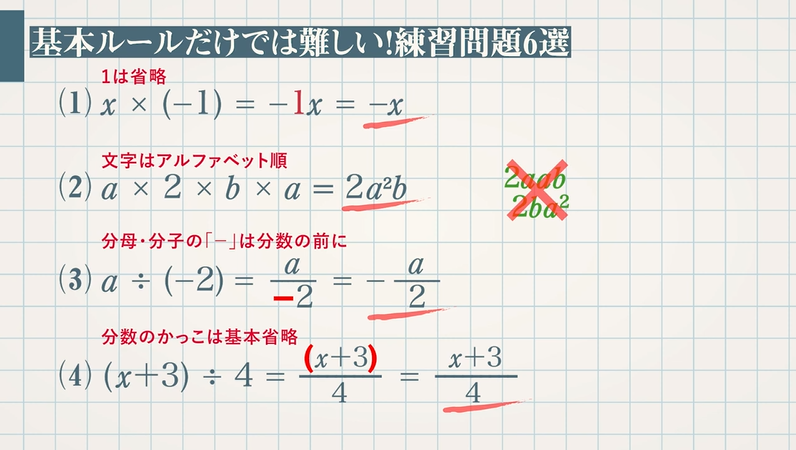
【問題】次の計算をしなさい。
\begin{eqnarray} \textcolor{green}{(1)}& &\textcolor{green}{x×(-1)}=-1x=\textcolor{red}{-x}\\ & &\textcolor{blue}{1 は省略}\\\\ \textcolor{green}{(2)}& &\textcolor{green}{a×2×b×a}=\textcolor{red}{2a^2b}\\ & &\textcolor{blue}{文字はアルファベット順}\\\\ \textcolor{green}{(3)}& &\textcolor{green}{a÷(-2)}=\frac{a}{-2}=\textcolor{red}{-\frac{a}{2}}\\ & &\textcolor{blue}{分母・分子の「-」は分数の前に}\\\\ \textcolor{green}{(4)}& &\textcolor{green}{(x+3)÷4}=\frac{(x+3)}{4}=\textcolor{red}{\frac{x+3}{4}}\\ & &\textcolor{blue}{分数のかっこは基本省略}\\\\ \end{eqnarray}

\begin{eqnarray}\textcolor{green}{(5)}& &\textcolor{green}{a÷b×c÷d}\\ & &\textcolor{blue}{除法は、乗法になおして計算(逆数にしてかける)}\\ & &=a×\frac{1}{b}×c×\frac{1}{d}=\textcolor{red}{\frac{ac}{bd}} \\\\\\ \textcolor{green}{(6)}& &\textcolor{green}{2×(n+1)-b÷c}\\ & &\textcolor{blue}{「+」「-」は省略できないので、前後にわける}\\ & &=2(n+1)-b×\frac{1}{c}=\textcolor{red}{2(n+1)-\frac{b}{c}} \end{eqnarray}
【問題】次の式を、$\textcolor{green}{×}$ や $\textcolor{green}{÷}$ の記号を使って表しなさい。
さきほどとは逆に、文字式のルールで表されたものを記号を使って表してみましょう。
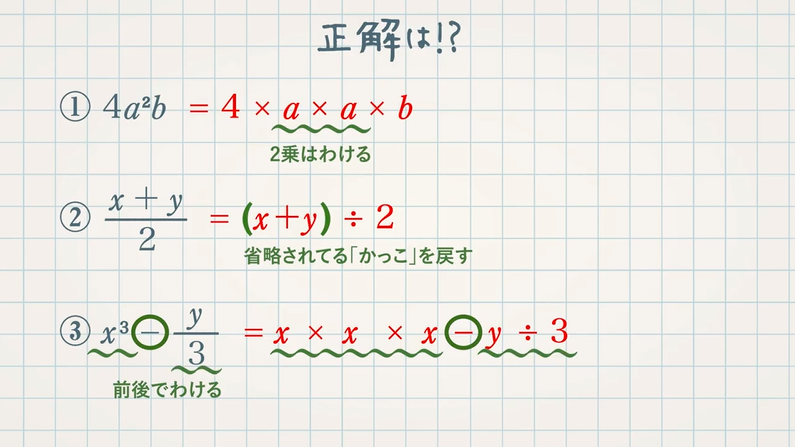
① $\textcolor{green}{4a^2b}=\textcolor{red}{4×a×a×b}$ $\textcolor{blue}{2}$ 乗もわける
② $\textcolor{green}{\frac{x+y}{2}}=\textcolor{red}{(x+y)÷2}$ 省略されているかっこを戻す
③ $\textcolor{green}{x^3-\frac{y}{3}}=\textcolor{red}{x×x×x-y÷3}$ 「$\textcolor{blue}{-}$」の前後でわける
コンテンツやシステムに関するお問い合わせ・オファーはこちらから