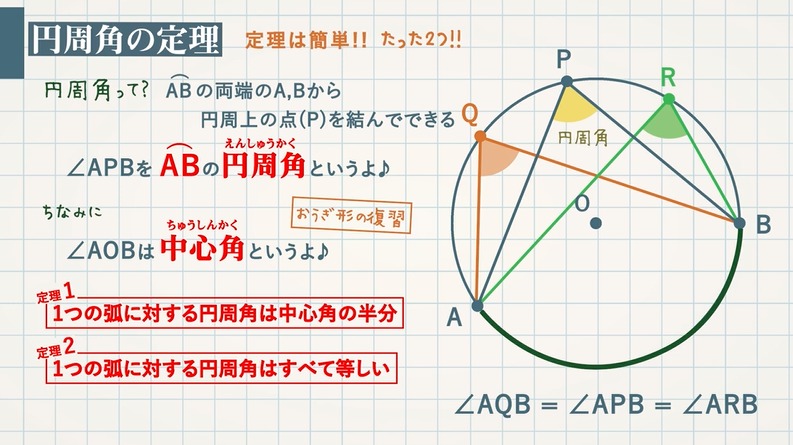
$\stackrel{\frown}{\rm AB}$ の両端 $\rm A, B$ から円周上の点($\rm P$) を結んでできる $\rm ∠APB$ を$\textcolor{blue}{\stackrel{\frown}{\rm AB}}$ の円周角といいます。また、$\rm ∠AOB$ は中心角といいます。(おうぎ形の復習)

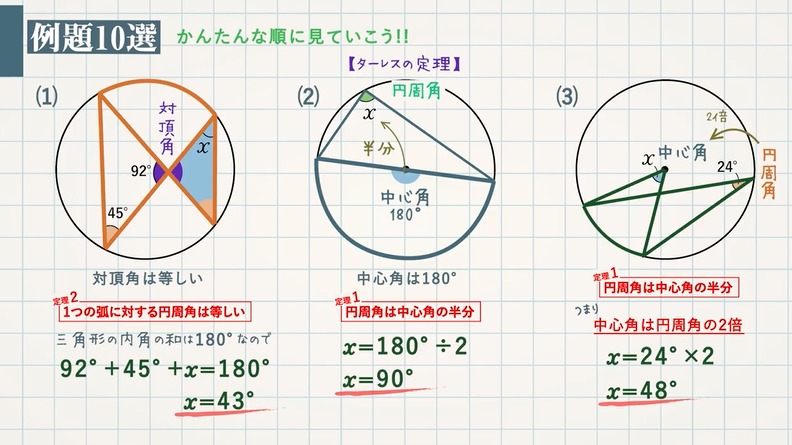
円周角の定理はたったの $2$ つだけです。簡単なので、しっかり確認しましょう。

定理 $\textcolor{blue}{1}$ ：$\textcolor{blue}{1}$ つの弧に対する円周角は中心角の半分

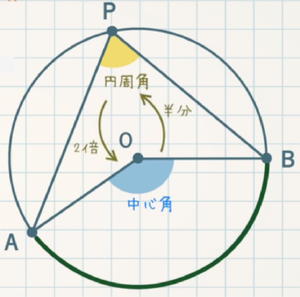
定理 $\textcolor{blue}{2}$ ：$\textcolor{blue}{1}$ つの弧に対する円周角はすべて等しい

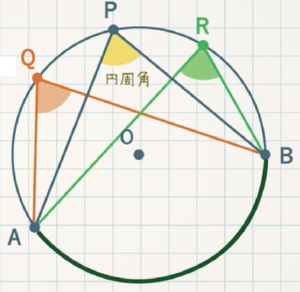
$\rm ∠AQB=∠APB=∠ARB$ (すべて $\stackrel{\frown}{\rm AB}$ に対する円周角)

【例題 $\textcolor{green}{10}$ 選】

(1) 対頂角は等しい(紫の角)

$\textcolor{blue}{1}$ つの弧(オレンジ色)に対する円周角は等しい

三角形の内角の和は $180°$ なので、

$92°+45°+x=180°$　$\textcolor{red}{x=43°}$

(2) 中心角は $\textcolor{blue}{180°}$

円周角は中心角の半分

$x=180°÷2$　 $\textcolor{red}{x=90°}$

(3) $\textcolor{blue}{x}$ は中心角

円周角は中心角の半分→中心角は円周角の $\textcolor{blue}{2}$ 倍

$x=24°×2$　 $\textcolor{red}{x=48°}$

(4) 弧(オレンジ色)の中心角は $\textcolor{blue}{360°-150°=210°}$

$x$ は弧(オレンジ色)の円周角なので、

$x=210÷2$ 　$\textcolor{red}{x=105°}$

(5) POINT：等しい弧に対する中心角・円周角は等しい

$\stackrel{\frown}{\rm AB}=\stackrel{\frown}{\rm BC}$ より、$\textcolor{blue}{\stackrel{\frown}{\rm AC}}$ の円周角を二等分　$\textcolor{blue}{38°÷2=19°}$ 

$x$ は $\stackrel{\frown}{\rm BC}$ (黄土色) に対する円周角。よって、$\textcolor{red}{x=19°}$

(6) 弧(緑色)に対する円周角は、$\textcolor{blue}{180°÷2=90°}$ 

弧(紫色)に対する円周角は、$\textcolor{blue}{12°}$

よって、$x=90°+12°$　$\textcolor{red}{x=102°}$

(7) 高校だと接弦定理と習います。

POINT：接点と中心を結ぶと接線と垂直になる

角(青色) $90°-77°=\textcolor{blue}{13°}$

POINT：半径 $\textcolor{blue}{2}$ 本と弦で二等辺三角形ができる

二等辺三角形の底角は等しいので、角(オレンジ色)は、$180°-13°-13°=\textcolor{blue}{154°}$

角(オレンジ色)は、弧(緑色)の中心角、$x$ は弧(緑色)の円周角なので、$x=154°÷2$　 $\textcolor{red}{x=77°}$

(8) $\textcolor{blue}{105°}$ は中心角ではない点に注意しましょう。

$\textcolor{blue}{x}$ は弧(青色)に対する円周角となります。

$61°+x+x$ (オレンジの角の合計) $=$ $105°$

これを解いて、$\textcolor{red}{x=22°}$ となります。

(9) $\textcolor{blue}{\stackrel{\frown}{\rm AF}}$ に対する中心角は、$\textcolor{blue}{6}$ 等分なので、$360°÷6=60°$ 

$\stackrel{\frown}{\rm AF}$ に対する円周角 $\rm ∠ABF=60°÷2=30°$ 

$\stackrel{\frown}{\rm BE}$ (青色) に対する円周角 $\rm ∠BAE=180°÷2=90°$ 

三角形の内角の和は $180°$ なので、$30°+90°+x=180°$ よって、$\textcolor{red}{x=60°}$

(10) POINT：接点と中心を結ぶと接線と垂直になる。

$\textcolor{blue}{\stackrel{\frown}{\rm BC}}$ に対する中心角 $\textcolor{blue}{\rm ∠BOC}$ は、四角形($\textcolor{blue}{\rm ABOC}$)の内角なので、$360°-90°-90°-64°=116°$ 

$\rm ∠BPC$ は $\stackrel{\frown}{\rm BC}$ に対する円周角なので、$116°÷2=58°$

$\rm ∠BOC=3$ つの角の合計なので、$30°+58°+x=116°$ 

よって、$\textcolor{red}{x=28°}$