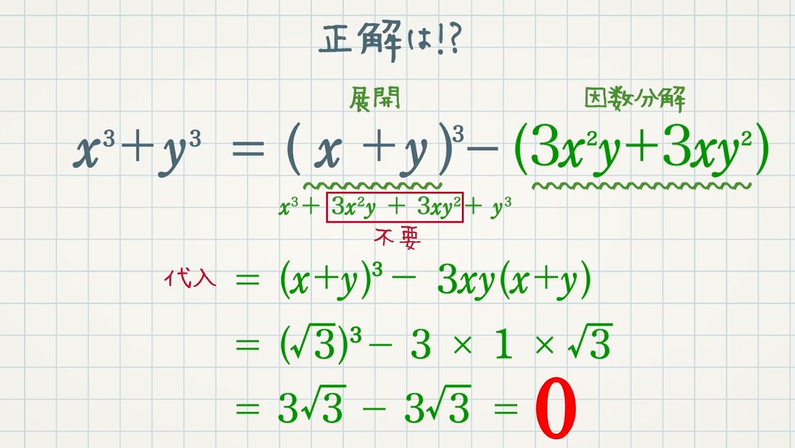
【例題】 $\textcolor{green}{x=\frac{1}{\sqrt{5}+\sqrt{3}}　y=\frac{1}{\sqrt{5}-\sqrt{3}}}$　のとき、次の式の値を求めなさい。

\begin{eqnarray} \textcolor{green}{(1)}& &\textcolor{green}{x+y}　→　代入して計算\\ &=&\frac{1}{\sqrt{5}+\sqrt{3}}+\frac{1}{\sqrt{5}-\sqrt{3}}\\\\ &=&\frac{(\sqrt{5}+\sqrt{3})+(\sqrt{5}-\sqrt{3})}{(\sqrt{5}+\sqrt{3})(\sqrt{5}-\sqrt{3})}=\frac{2\sqrt{5}}{2}=\textcolor{red}{\sqrt{5}} \end{eqnarray}

\begin{eqnarray} \textcolor{green}{(2)}& &\textcolor{green}{xy}　代入して計算\\ &=&\frac{1}{\sqrt{5}+\sqrt{3}}×\frac{1}{\sqrt{5}-\sqrt{3}}\\\\ &=&\frac{1}{(\sqrt{5}+\sqrt{3})(\sqrt{5}-\sqrt{3})}=\textcolor{red}{\frac{1}{2}} \end{eqnarray}

(3)～(5)は先ほど求めた $\textcolor{blue}{x+y=\sqrt{5} , xy=\frac{1}{2}}$ を代入して計算します。

\begin{eqnarray} \textcolor{green}{(3)　x^2+y^2}=(x+y)^2-2xy=\textcolor{Red}{4} \end{eqnarray}

\begin{eqnarray} \textcolor{green}{(4)　x^y+xy^2}=xy(x+y) =\frac{1}{2}×(\sqrt{5})=\textcolor{red}{\frac{\sqrt{5}}{2}} \end{eqnarray}

\begin{eqnarray}\textcolor{green}{ (5)　\frac{1}{x}+\frac{1}{y}}=\frac{\sqrt{5}}{\frac{1}{2}}=\sqrt{5}÷\frac{1}{2}=\textcolor{red}{2\sqrt{5}} \end{eqnarray}

【問題①】 $\textcolor{green}{x+y=\sqrt{3} , xy=1}$ のとき、次の式の値を求めなさい。

\begin{eqnarray}&　&\textcolor{green}{x^3+y^3}\\\\ &=&(x+y)^3-(3x^2y+3xy^2)\\\\ &=&(\textcolor{blue}{x+y})^3-3\textcolor{blue}{xy}(\textcolor{blue}{x+y})　x+y=\sqrt{3} , xy=1を代入\\\\ &=&(\sqrt{3})^3-3×1×\sqrt{3}\\ \\&=&3\sqrt{3}-3\sqrt{3}=\textcolor{red}{0} \end{eqnarray}

【問題②】 $\textcolor{green}{\sqrt{3}=1.7321}$ のとき、次の式の値を求めなさい。

\begin{eqnarray} &　&\textcolor{green}{\frac{\sqrt{3}}{\sqrt{3}-1}}\\\\ &=&\frac{\sqrt{3}×(\sqrt{3}+1)}{\sqrt{3}-1×(\sqrt{3}+1)}　有理化\\\\ &=&\frac{\sqrt{3}(\sqrt{3}+1)}{(\sqrt{3}-1)(\sqrt{3}+1)}\\\\ &=&\frac{3+\sqrt{3}}{3-1}\\\\ &=&\frac{3+\sqrt{3}}{2}　\sqrt{3}=1.7321を代入\\\\ &=&\frac{4.7321}{2}=\textcolor{red}{2.36605} \end{eqnarray}