等式の変形とは、等式の性質を使って変形して、指定された文字について解くことをいいます。

等式変形は、いろいろな単元の問題や解説に出てくるので、しっかり確認していきましょう。

例えば、$2x+y=5$ ( $2$ 元 $1$ 次方程式) を $\textcolor{blue}{y}$ について解くと 、$\textcolor{blue}{y=-2x+5}$ ( $1$ 次関数) になります。

等式の性質と移項について確認しておきましょう。

$\rm A+C=B$ という式を $\rm A=□$ と変形する($\rm A$について解く)とき、

\begin{eqnarray} \rm A+C&=&\rm B　　 　・・・①\\ \rm A+C\textcolor{blue}{-\rm C}&=&\rm B\textcolor{blue}{\rm -C}　・・・②\\ \rm A&=&\rm B\textcolor{blue}{-\rm C}　・・・③ \end{eqnarray}

①と③の式を比べると、$+\rm C$ の符号が変わって($-\rm C$ が)他方の辺に移っていることがわかります。このことを移項 (項を他辺に移す) といいます。

【実践】 次の等式を〔　〕の中の文字について解きなさい。

\begin{eqnarray} \textcolor{green}{(1)　　2m+n}&\textcolor{green}{=}&\textcolor{green}{3 〔n〕}　2m を移項する\\ \textcolor{red}{n}&\textcolor{red}{=}&\textcolor{red}{3-2m}\\ \end{eqnarray} \begin{eqnarray} \\\textcolor{green}{(2)　3x-6y}&\textcolor{green}{=}&\textcolor{green}{18 〔y〕}　3x を移項する\\\\ -6y&=&\textcolor{blue}{-3x}+18　両辺を -6 でわる\\\\ y&=&\frac{3x-18}{6}　3 で約分\\\\ \textcolor{red}{y}&\textcolor{red}{=}&\textcolor{red}{\frac{x-6}{2}}　{\rm or}　\textcolor{red}{y=\frac{1}{2}x-3} \end{eqnarray}

次の(3)～(6)はすべて図形の公式となっています。アルファベットの意味はだいたい決まっていて、$\textcolor{blue}{\rm V=}$ 体積、$\textcolor{blue}{h=}$ 高さ、$\textcolor{blue}{ℓ=}$ 周、$\textcolor{blue}{\rm S=}$ 面積、$\textcolor{blue}{r=}$ 半径　などがあります。

\begin{eqnarray} &(3)&　{\rm V}=\frac{1}{3}a^2h〔h〕正四角錐の体積\\\\ &(4)&　ℓ=2(a+b)〔a〕長方形の周の長さ\\\\ &(5)&　{\rm S}=\frac{1}{2}ah〔h〕三角形の面積\\\\ &(6)&　{\rm S}=\frac{(a+b)h}{2}〔a〕台形の面積 \end{eqnarray}

【実践】次の等式を〔　〕の中の文字について解きなさい。

\begin{eqnarray} \textcolor{green}{(3)　\rm {V}}& \textcolor{green}{=}& \textcolor{green}{\frac{1}{3}a^2h} \textcolor{green}{〔h〕}　\textcolor{blue}{両辺を入れかえる} \\\\ \frac{1}{3}a^2h&=&\rm V　　両辺に 3 をかける\\\\ a^2h&=&3\rm V　　両辺を a^2 でわる\\\\ \textcolor{red}{h}&\textcolor{red}{=}&\textcolor{red}{\frac{3\rm V}{a^2}} \end{eqnarray}

求めたい文字が右側にあるときは両辺を入れかえます。

\begin{eqnarray}\textcolor{green}{(4) 　　ℓ}&\textcolor{green}{=}&\textcolor{green}{2(a+b)〔a〕}　両辺を入れかえる\\\\ 2(a+b)&=&ℓ　両辺を 2 でわる\\\\ a+b&=&\frac{ℓ}{2}　b を移項する\\\\ \textcolor{red}{a}&\textcolor{red}{=}&\textcolor{red}{\frac{ℓ}{2}-b} \end{eqnarray}

よくある答えの間違いとして、下のものがあるので注意しましょう。

\begin{eqnarray} 　　a&=&\frac{ℓ\textcolor{blue}{-b}}{2}←✕　　a&=&\frac{ℓ\textcolor{blue}{-2b}}{2}←こっちなら〇 \end{eqnarray}

\begin{eqnarray} \textcolor{green}{(6)　　\rm S}& \textcolor{green}{=}& \textcolor{green}{\frac{(a+b)h}{2}〔a〕}　両辺を入れかえ 2 をかける\\\\ (a+b)h&=&2{\rm S}　両辺を h でわる\\\\ a+b&=&\frac{2\rm S}{h}　b を移項する\\\\ \textcolor{red}{a}&\textcolor{red}{=}&\textcolor{red}{\frac{2\rm S}{h}-b} \end{eqnarray}

等式の変形では、形が違っても正解の場合があるので注意

\begin{eqnarray} \textcolor{red}{a=\frac{2{\rm S}-bh}{h}}　{\rm or}　\textcolor{red}{a=-b+\frac{2\rm S}{h}} 　でも\rm OK\end{eqnarray}

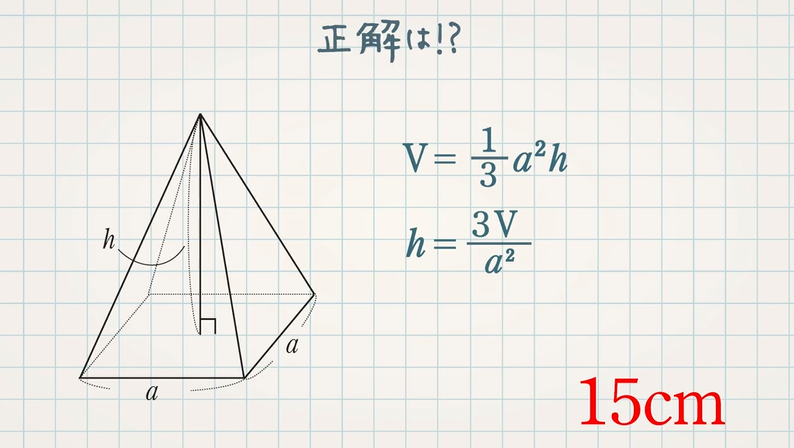
【問題】 $\textcolor{green}{a}$ ( $\textcolor{green}{1}$ 辺) の長さが $\textcolor{green}{2\rm cm}$ 、$\textcolor{green}{\rm V}$ (体積) が $\textcolor{green}{20}$ $\textcolor{green}{\rm cm^2}$ のとき $\textcolor{green}{h}$ (高さ) を求めなさい。

正四角錐の体積の求め方は、${\rm V}=\frac{1}{3}a^2h$ でした。高さを求めたいので、$h$ についての式に変形すると、

\begin{eqnarray} h&=&\frac{3\rm V}{a^2}　　{\rm V},a にそれぞれの数値を代入すると\\\\ h&=&\frac{3×20}{2^2}\\\\ h&=&\textcolor{red}{15 \rm cm} \end{eqnarray}