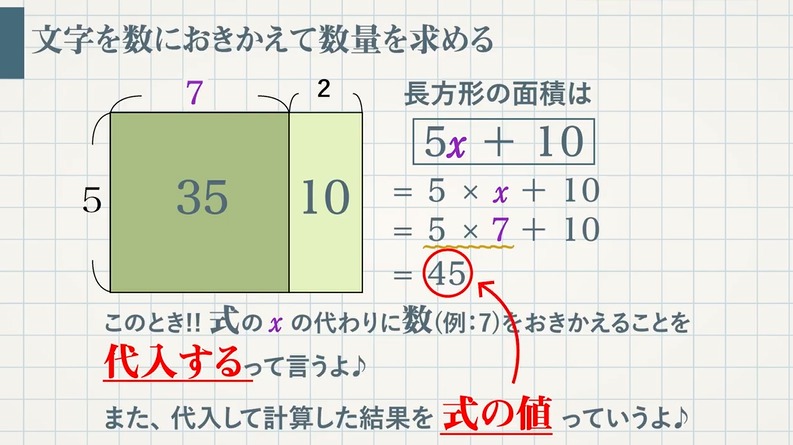
縦の長さが $5$、横の長さが $x+2$ の長方形の面積は $\textcolor{blue}{5x+10}$ と表すことができます。

今、式の $\textcolor{blue}{x}$ の代わりに $\textcolor{blue}{7}$ をあてはめて計算すると、

\begin{eqnarray}   &　&5\textcolor{blue}{x}+10\\ &=&5×\textcolor{blue}{7}+10\\ &=&35+10\\ &=&45 \end{eqnarray}

このとき、式の $x$ の代わりに数(例：$7$) をあてはめることを、$x$ に $7$ を代入するといい、また代入して計算した結果 $45$ を $x=7$ のときの式の値といいます。

【練習問題】$\textcolor{green}{x=3,y=-2}$ のとき、次の式の値を求めなさい。

\begin{eqnarray} \textcolor{green}{(1)}& &\textcolor{green}{11-3x}=11-3×\textcolor{blue}{3}=\textcolor{red}{2}\\\\\textcolor{green}{ (2)}& &\textcolor{green}{\frac{24}{y}}=24÷\textcolor{blue}{(-2)}=\textcolor{red}{-12}\\\\\textcolor{green}{(3)}& &\textcolor{green}{-3x^2}=-3×\textcolor{blue}{3}×\textcolor{blue}{3}=\textcolor{red}{-27}\\\\ \textcolor{green}{(4)}& &\textcolor{green}{-\frac{1}{3}x-4y}=-\frac{1}{3}×\textcolor{blue}{3}-4×\textcolor{blue}{(-2)}=\textcolor{red}{7} \end{eqnarray}

【問題】気温 $\textcolor{green}{t}$℃のときの音の速さを $\textcolor{green}{331.5+0.6 t}$ ($\textcolor{green}{\rm m}$/秒) とするとき、気温 $\textcolor{green}{30}$℃で、雷が光ってから $\textcolor{green}{2}$ 秒後に音が聞こえました。雷までの距離を求めなさい。

$\textcolor{blue}{t=30}$ なので、式の $\textcolor{blue}{t}$ に $\textcolor{blue}{30}$ を代入します。

\begin{eqnarray} &　&331.5+0.6×\textcolor{blue}{30}\\ &=&331.5+18\\ &=&\textcolor{red}{349.5(\rm m/秒)}\\\\ &速さ&×時間=距離なので、\\ & &349.5×2=\textcolor{red}{699\rm m} \end{eqnarray}