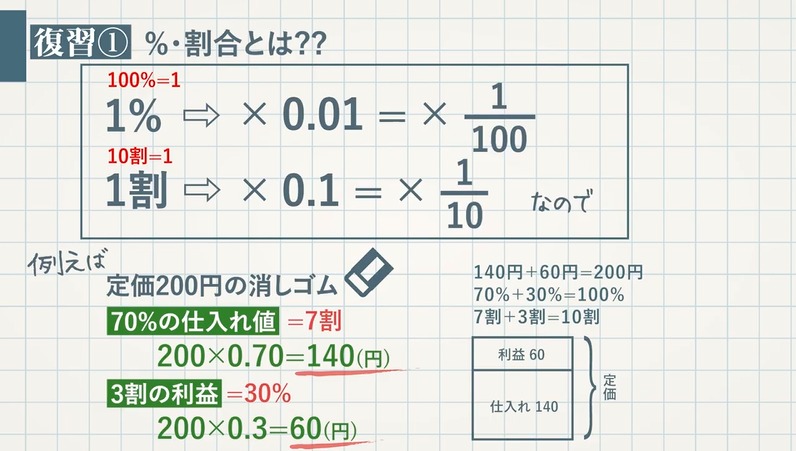
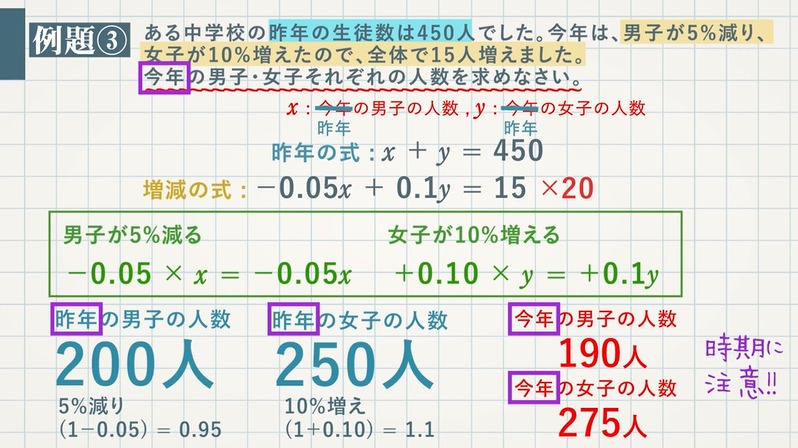
復習として％や割合について確認しておきましょう。

$\textcolor{blue}{100}$ % $\textcolor{blue}{=1}$ なので、 $1$ % → $×0.01= ×\frac{1}{100}$

$\textcolor{blue}{10}$ 割 $\textcolor{blue}{=1}$ なので、$1$ 割 → $×0.1=×\frac{1}{10} $

例えば、定価 $200$ 円の消しゴムの、

$\textcolor{blue}{70}$ % の仕入れ値→ $\textcolor{blue}{7}$ 割　$200×\textcolor{blue}{0.70}=140$ (円)

$\textcolor{blue}{3}$ 割の利益→$\textcolor{blue}{30}$ %　$ 200×\textcolor{blue}{0.3}=60$ (円) 

定価 $\textcolor{green}{200}$ 円の消しゴムの $\textcolor{green}{2}$ 割引きの値段は？

$2$ 割引き→ $10$ 割 $-$ $2$ 割 $=$ $\textcolor{blue}{8}$ 割 ($\textcolor{blue}{0.8}$) なので、

\begin{eqnarray} & &200×(1-0.2)\\ &=&200×\textcolor{blue}{0.8}\\ &=&\textcolor{red}{160(円)} \end{eqnarray}

定価 $\textcolor{green}{200}$ 円の消しゴムの $\textcolor{green}{35}$ %offの値段は？

$35$ %off → $100$ % $-$ $35$ % $=$ $\textcolor{blue}{65}$ % ($\textcolor{blue}{0.65}$)なので、

\begin{eqnarray} & &200×(1-0.35)\\ &=&200×\textcolor{blue}{0.65}\\ &=&\textcolor{red}{130(円)} \end{eqnarray}

増加・上乗せ（税込み等）はどう表せるでしょうか。

(例 $\textcolor{green}{1}$ )　$\textcolor{green}{200}$ 人の部員が $\textcolor{green}{15}$ % 増える

\begin{eqnarray} 200×\textcolor{blue}{1.15}=\textcolor{red}{230(人)} \end{eqnarray}

(例 $\textcolor{green}{2}$ )　$\textcolor{green}{500}$ 円の税込み ($\textcolor{green}{10}$ %) 価格

\begin{eqnarray} 500×\textcolor{blue}{1.10}=\textcolor{red}{550(円)} \end{eqnarray}

(例 $\textcolor{green}{3}$ )　仕入れ値 ($\textcolor{green}{300}$円) に $\textcolor{green}{2}$ 割の利益を乗せる

\begin{eqnarray} 300×\textcolor{blue}{1.2}=\textcolor{red}{360(円)} \end{eqnarray}

【例題①】定価の合計が $\textcolor{green}{900}$ 円のカレーとサラダを注文したところ、カレーが $\textcolor{green}{1}$ 割引き、サラダ $\textcolor{green}{2}$ 割引きのセット価格が $\textcolor{green}{785}$ 円となりました。カレーの定価とサラダの定価を求めなさい。

カレーの定価を $x$ 円、サラダの定価を $y$ 円とすると、カレーは $1$ 割引きなので $\textcolor{blue}{0.9x}$、サラダは $2$ 割引きなので$\textcolor{blue}{0.8y}$ となります。

 \begin{eqnarray} 定価の式：x+y&=&900\\ セット価格の式：0.9x+0.8y&=&785 \end{eqnarray}

 \begin{cases} x + y = 900 & \\ 0.9x + 0.8y = 785 & \end{cases} 

これを解くと、$\textcolor{blue}{(x , y)=(650 , 250)}$

カレーの定価　$\textcolor{red}{650}$ 円

サラダの定価　$\textcolor{red}{250}$ 円

【例題②】定価の合計が $\textcolor{green}{5200}$ 円のシャツとズボンを、シャツ $\textcolor{green}{20}$ %引き、ズボン $\textcolor{green}{30}$ %引きのセール価格 $\textcolor{green}{3840}$ 円で購入しました。シャツとズボンそれぞれの定価を求めなさい。

シャツの定価を $x$ 円、ズボンの定価を $y$ 円とすると、シャツは $20$ %引きなので $\textcolor{blue}{0.8x}$、ズボンは $30$ %割引きなので $\textcolor{blue}{0.7y}$ となります。

\begin{eqnarray} 定価の式：x+y&=&5200\\ セール価格の式：0.8x+0.7y&=&3840\end{eqnarray}

\begin{cases} x + y = 5200 & \\ 0.8x + 0.7y = 3840 & \end{cases} 

これを解くと、$\textcolor{blue}{(x , y)=(2000 , 3200)}$

シャツの定価　$\textcolor{red}{2000}$ 円

ズボンの定価　$\textcolor{red}{3200}$ 円

【例題③】ある中学校の昨年の生徒数は $\textcolor{green}{450}$ 人でした。今年は、男子が $\textcolor{green}{5}$ %減り、女子が $\textcolor{green}{30}$ %増えたので、全体で $\textcolor{green}{15}$ 人増えました。今年の男子・女子の人数を求めなさい。

昨年の男子の人数を $x$ 人、女子の人数を $y$ 人とすると、今年の男子は $5$ %減ったので $\textcolor{blue}{-0.05x}$、今年の女子は $10$ %増えたので $\textcolor{blue}{0.1y}$ となります。

\begin{eqnarray} 昨年の人数の式：x+y&=&450\\  今年の人数増減の式：-0.05x+0.1y&=&15\end{eqnarray}

\begin{cases} x + y = 450 & \\ -0.05x + 0.1y = 15 & \end{cases} 

これを解くと、$\textcolor{blue}{(x , y)=(200 , 250)}$

よって、今年の男子・女子の人数は

\begin{eqnarray} 男子：190×0.95=\textcolor{red}{190 人}\\ 女子：250×1.10=\textcolor{red}{275 人} \end{eqnarray}