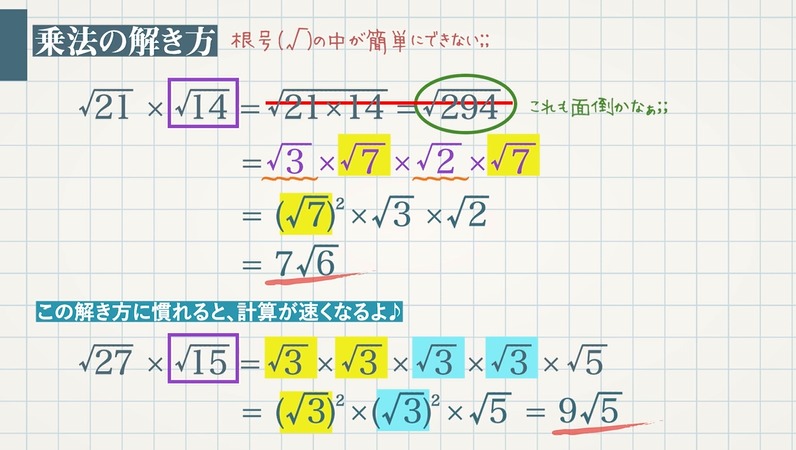
平方根の乗法の解き方は、数字どうし、$\sqrt{　}$ どうしをかけます。

【例題】次の計算をしなさい。

\begin{eqnarray} &　&\textcolor{green}{2\sqrt{5}×4\sqrt{3}}\\ &=&(2×\sqrt{5})×(4×\sqrt{3}) \\ &=&2×4×\sqrt{5}×\sqrt{3}\\ &=&\textcolor{red}{8\sqrt{15}} \end{eqnarray}

こんな風に出題されても、答えは同じになります。

\begin{eqnarray} & &\textcolor{green}{\sqrt{20}×\sqrt{48}}\\ &=&\sqrt{\textcolor{blue}{2^2}×5}×\sqrt{\textcolor{blue}{2^2}×\textcolor{blue}{2^2}×3}\\ &=&2\sqrt{5}×4\sqrt{3}　\\&=&\textcolor{red}{8\sqrt{15}} \end{eqnarray}

ポイント:根号の中の数はなるべく小さな自然数に

この他にも根号をふくむ乗法の計算を早くする方法があります。確認しておきましょう。

【例題】次の計算をしなさい。

\begin{eqnarray} &　&\textcolor{green}{\sqrt{21}×\sqrt{14}}　そのまま計算しない\\ &=&\textcolor{blue}{\sqrt{7}}×\sqrt{3}×\textcolor{blue}{\sqrt{7}}×\sqrt{2}\\ &=&\textcolor{blue}{(\sqrt{7})^2}×\sqrt{3}×\sqrt{2}\\ &=&\textcolor{red}{7\sqrt{6}}\\\\\\ &　&\textcolor{green}{\sqrt{27}×\sqrt{15}}　そのまま計算しない\\&=&\textcolor{blue}{\sqrt{3}}×\textcolor{blue}{\sqrt{3}}×\textcolor{blue}{\sqrt{3}}×\textcolor{blue}{\sqrt{3}}×\sqrt{5}\\ &=&\textcolor{blue}{(\sqrt{3})^2}×\textcolor{blue}{(\sqrt{3})^2}×\sqrt{5}\\ &=&\textcolor{red}{9\sqrt{5}} \end{eqnarray}

除法も乗法と同じく、数字どうし、$\sqrt{　}$ どうしをわります。

【例題】次の計算をしなさい。

\begin{eqnarray} \textcolor{green}{6\sqrt{10}÷3\sqrt{2}}&=&\frac{6\sqrt{10}}{3\sqrt{2}}=\textcolor{red}{2\sqrt{5}} \end{eqnarray}

除法の計算の結果は、ふつう分母を有理化して、分母に根号がふくまない形にしておきます。

\begin{eqnarray}\textcolor{green}{  \sqrt{2}÷\sqrt{3}}&=&\frac{\sqrt{2}}{\sqrt{3}}　分母の有理化\\\\ &=&\frac{\sqrt{2}\textcolor{blue}{×\sqrt{3}}}{\sqrt{3}\textcolor{blue}{×\sqrt{3}}}=\textcolor{red}{\frac{\sqrt{6}}{3}} \end{eqnarray}